Chapter 1

Figure 1.1: Aristotle, circa 384-322 BC

Source: http://www.departments.bucknell.edu/history/carnegie/aristotle/bust.html

Figure 1.2: The Christian Aristotelian cosmos, engraving from Peter Apian's Cosmographia, 1524

Source: http://abyss.uoregon.edu/~js/glossary/ptolemy.html

Figure 1.3: Ptolemy, AD 127-145

Source: http://galileo.rice.edu/sci/theories/ptolemaic_system.html

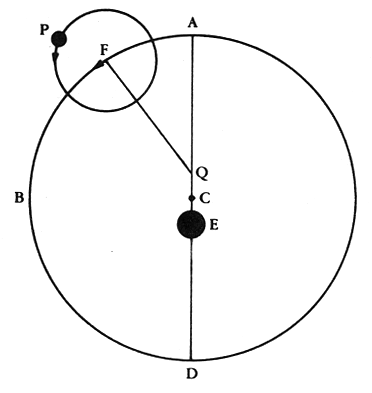
Figure 1.4: A ptolomaic construction of planetary motion, where E is the Earth, C the geometric center of the eccentric circle, Q the equant point, F the center of the epicycle, and P the planet.

Source: http://galileo.rice.edu/sci/theories/ptolemaic_system.html, From Michael J. Crowe, Theories of the World from Antiquity to the Copernican Revolution.

Figure 1.5: Nicolaus Copernicus (Mikolaj Kopernik), 1473-1643

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Copernicus.html

First Epicycle

Second Epicycle

Center

Earth

Equant

Deferent

Celestial Object

Figure 1.6: Example of elliptical (first epicycle) and retrograde (second epicycle) orbits produced using epicycles.

Figure 1.7: Isaac Newton (1643 - 1727)

From a portrait by Kneller in 1689

Source:

http://www-history.mcs.st-andrews.ac.uk/history/PictDisplay/Newton.html

Figure 1.8: Albert Einstein (1879 – 1955)

Source:

http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Einstein.html

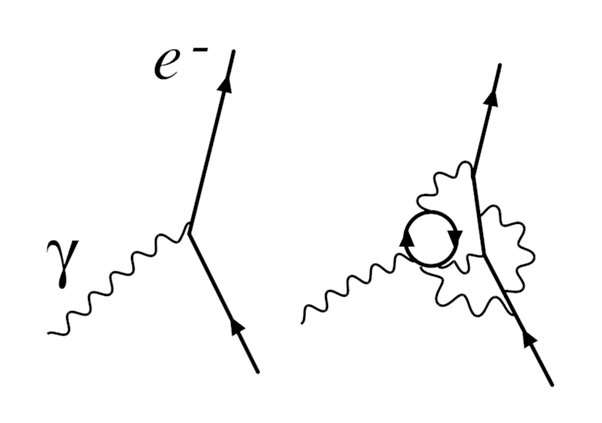
Figure 1.9: Renormalization in quantum electrodynamics: A single interaction (left) between a photon (g) and electron (e^-) is replaced by multiple interactions (right).

Source: http://en.wikipedia.org/wiki/Renormalization#A_loop_divergence

Figure 1.10: Emmy Noether (1882-1935)

Source: www-gap.dcs.st-and.ac.uk/~history/Biographies/Noether_Emmy.html

Chapter 2

Figure 2.1 : Christian Huygens, 1629 - 1695
Source: http://www-history.mcs.st-and.ac.uk/history/PictDisplay/Huygens.html

Check with SPL/Photo Researchers (b/w vs. color image)

Figure 2.2: Thomas Young, 1773 - 1829

Source:

http://www-history.mcs.st-andrews.ac.uk/Mathematicians/Young_Thomas.html

Figure 2.3: Augustin Fresnel, 1788 - 1827

Source:

http://www-history.mcs.st-andrews.ac.uk/history/PictDisplay/Fresnel.html

Figure 2.4: George Gabriel Stokes, 1819 – 1903

Source:

http://www-history.mcs.st-andrews.ac.uk/PictDisplay/Stokes.html

Figure 2.5: James MacCullagh, 1809 - 1847

Source: http://www-history.mcs.st-andrews.ac.uk/history/PictDisplay/MacCullagh.html

Figure 2.6: Joseph Boussinesq, 1842-1929

Source: http://ambafrance-ca.org/HYPERLAB/PEOPLE/bouss.html

Figure 2.7: William Thomson (Lord Kelvin), 1824 - 1907

Source:

http://www-history.mcs.st-andrews.ac.uk/history/PictDisplay/Thomson.html

Figure 2.8: James Clerk Maxwell, 1831 - 1879

Source:

http://www-history.mcs.st-and.ac.uk/history/Mathematicians/Maxwell.html

Figure 2.9: Albert Michelson, 1852-1931

Source:

http://nobelprize.org/nobel_prizes/physics/laureates/1907/index.html

Figure 2.10: Hendrik Lorentz, 1853 - 1928

Source: http://www-history.mcs.st-andrews.ac.uk/history/PictDisplay/Lorentz.html

Figure 2.11: Jules Henri Poincare, 1854 - 1912

Source :

http://www-history.mcs.st-and.ac.uk/history/PictDisplay/Poincare.html

Figure 2.12: Max Planck, 1858 - 1947

Source:

http://www-gap.dcs.st-and.ac.uk/~history/Biographies/Planck.html

Figure 2.13: Neils Bohr, 1885-1962

Source:

http://www-gap.dcs.st-and.ac.uk/~history/Biographies/Bohr_Niels.html

Figure 2.14: Louis Victor de Broglie, 1892-1987

Source: http://www-gap.dcs.st-and.ac.uk/~history/PictDisplay/Broglie.html

Figure 2.15: Clinton Davisson and Lester Germer in 1927

Source: http://faculty.rmwc.edu/tmichalik/davisson.htm

(a) (b)

Figure 2.16: Images of diffraction from crystals of (a) x-rays and (b) electrons of similar wavelength

Source: http://online.cctt.org/physicslab/content/phyapb/lessonnotes/dualnature/Davisson_Germer.asp;

Copyright © 1997-2002 Catharine H. Colwell. All rights reserved. PhysicsLAB.

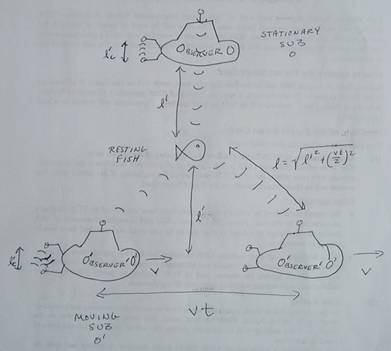
Figure 2.17: Time Dilation: The clock on O¢ ticks slower than the clock on O by the factor because waves travel farther between transmission and detection. Both O and O¢ measure the same number of clock cycles for a wave to propagate from their own sub to the fish and back. Hence they agree on distances perpendicular to the direction of relative motion.

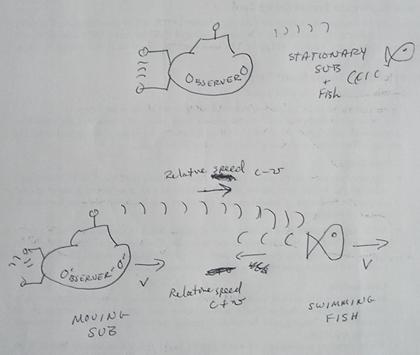
Figure 2.18: Length Contraction: The true wave propagation time for the co-moving sub and fish is longer than for the stationary sub and fish by the factor 1/(1-v²/c_s²). Since the moving clock runs slow, the perceived propagation time is longer only by the factor . Hence the stationary sub observes a shorter length than the moving sub.

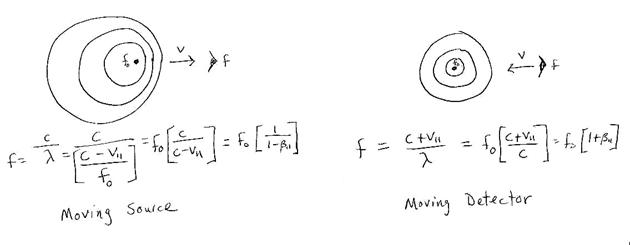
Figure 2.19: Classical Doppler shifts for moving (approaching) source and detector differ by a factor of . This factor is not affected by reversal of the velocity direction.

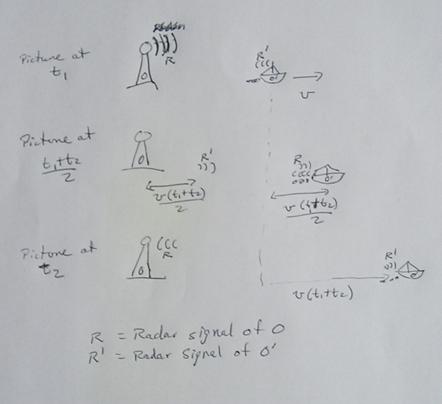
Figure 2.20: Velocity Measurement: Radar signals sent simultaneously by O and O¢ will also be received simultaneously after reflection. Although O¢’s clock ticks slowly, the proportionality between radar pulse propagation time and total time elapsed is the same as for O. Therefore both O and O¢ measure the same relative velocity.

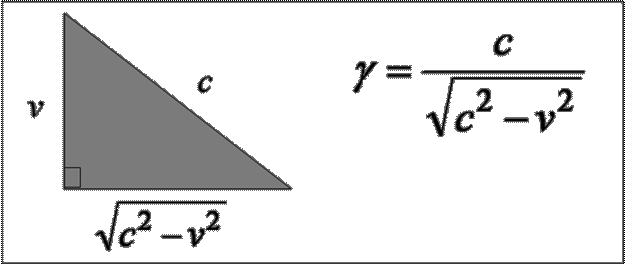
Figure 2.21: Energy, momentum, and mass have the same Pythagorean relation as light speed (c), velocity (u), and , respectively (with appropriate unit normalization).

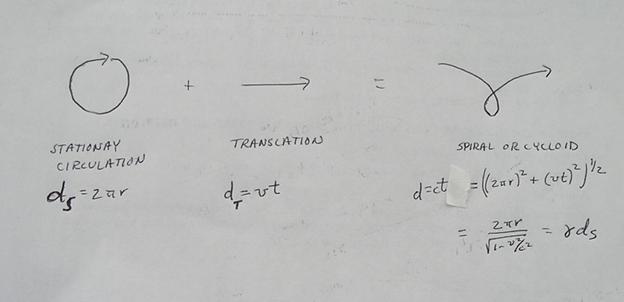
Figure 2.22: Time Dilation: Moving matter waves propagate farther than stationary matter waves during each cycle. Therefore moving clocks tick more slowly than stationary clocks. d_S = distance traveled in one cycle of stationary wave, d_T = translational distance. The distance formula for the cycloid is exact only for an integer number of cycles.

Chapter 3

Figure 3.1: J.J. Thomson, 1856-1940

Source: http://nobelprize.org/nobel_prizes/physics/laureates/1906/thomson-bio.html

Figure 3.2: Ernest Rutherford, 1871-1937

Source: http://nobelprize.org/nobel_prizes/chemistry/laureates/1908/rutherford-bio.html

Figure 3.3: Arnold J.W. Sommerfeld, 1868-1951

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Sommerfeld.html

Figure 3.4: Werner Heisenberg, 1901-1976

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Heisenberg.html

Figure 3.5: Erwin Schrodinger, 1887-1961

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Schrodinger.html

Figure 3.6: Wolfgang Pauli, 1900-1958

Source: http://www-history.mcs.st-andrews.ac.uk/history/PictDisplay/Pauli.html

Figure 3.7: Paul Dirac, 1902-1984

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Dirac.html

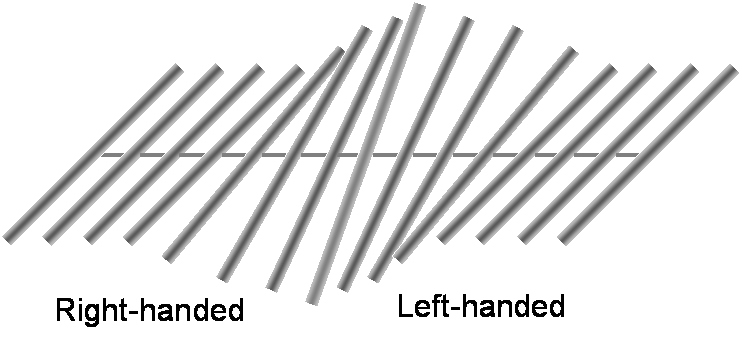
Figure 3.8: Rotation of a single bar on a torsion wave machine results in mirror-symmetric waves propagating in opposite directions. This is a one-dimensional analogue of production of particles and anti-particles. Matter and anti-matter are also always produced in mirror-symmetric pairs.

Chapter 4

Isaac Newton, 1643 – 1727

Source: http://www-groups.dcs.st-and.ac.uk/~history/Mathematicians/Newton.html

Pierre-Simon Laplace, 1749-1827

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Laplace.html

Lóránd Baron von Eötvös, 1848-1919

Source: http://www-groups.dcs.st-and.ac.uk/~history/Biographies/Eotvos.html

Albert Einstein, 1879 – 1955

Source: http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Einstein.html

David Hilbert, 1862 - 1943

http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Hilbert.html

Karl Schwarzschild, 1873-1916

http://www-groups.dcs.st-and.ac.uk/~history/PictDisplay/Schwarzschild.html

________________________________________________________________

http://www-history.mcs.st-and.ac.uk/history/Miscellaneous/Copyright.html

Copyright information

We do not own the copyright to the images used on this website.

We believe that most of the images are in the public domain and that provided you use them on a website you are unlikely to encounter any difficulty.

However, if you wish to use them in any other way -- in "paper" publishing or on a CD for example -- we cannot guarantee that there may not be outstanding copyright problems.

We have not kept a record of where we found any of the images we have used.

If you believe that you own the rights to any of the images we use, please contact us and we will either withdraw that picture or add an acknowledgement.

JOC/EFR August 2001

__________________________________________________________________--

Figure: Rotation of a single bar on a torsion wave machine results in mirror-symmetric waves propagating in opposite directions. This is a one-dimensional analogue of production of particles and anti-particles. Anti-matter and matter behave exactly as if they are mirror images of each other.